
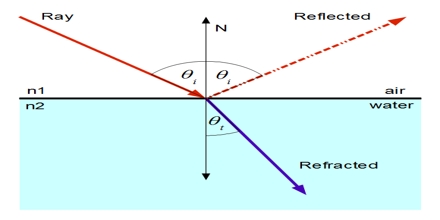
Thomas Young performed a celebrated experiment in 1803 demonstrating interference from two closely spaced slits. James Gregory (1638–1675) observed the diffraction patterns caused by a bird feather, which was effectively the first diffraction grating to be discovered. Isaac Newton studied these effects and attributed them to inflexion of light rays. The results of Grimaldi's observations were published posthumously in 1665. The effects of diffraction of light were first carefully observed and characterized by Francesco Maria Grimaldi, who also coined the term diffraction, from the Latin diffringere, 'to break into pieces', referring to light breaking up into different directions. Thomas Young's sketch of two-slit Diffraction for water waves, which he presented to the Royal Society in 1803. In this case, when the waves pass through the gap they become semi-circular. Diffraction is greatest when the size of the gap is similar to the wavelength of the wave.

The amount of diffraction depends on the size of the gap. Furthermore, quantum mechanics also demonstrates that matter possesses wave-like properties, and hence, undergoes diffraction (which is measurable at subatomic to molecular levels). These effects also occur when a light wave travels through a medium with a varying refractive index, or when a sound wave travels through a medium with varying acoustic impedance – all waves diffract, including gravitational waves, water waves, and other electromagnetic waves such as X-rays and radio waves. If there are multiple, closely spaced openings (e.g., a diffraction grating), a complex pattern of varying intensity can result.
This is due to the addition, or interference, of different points on the wavefront (or, equivalently, each wavelet) that travel by paths of different lengths to the registering surface. The characteristic bending pattern is most pronounced when a wave from a coherent source (such as a laser) encounters a slit/aperture that is comparable in size to its wavelength, as shown in the inserted image. In classical physics, the diffraction phenomenon is described by the Huygens–Fresnel principle that treats each point in a propagating wavefront as a collection of individual spherical wavelets. The MIKE 21's EMS module had a faster computing pace, but no output for wave directions and was incapable of including tidal current effects were the major drawbacks.Infinitely many points (three shown) along length d project phase contributions from the wavefront, producing a continuously varying intensity θ on the registering plate. Regarding the simulation of the passing-through boundary, the PMH model was better because of the nearly exact solution for this boundary. The EMS module for Mike 21 was slightly different than the previous three. The next three models (RDE, PBCG, and PMH) all performed very well on the simulation of wave shoaling, refraction, and diffraction, and they practically provided the same results for the case study presented. The RCPWAVE had accuracy problems in both wave height and direction. The Ref/Dif-1 had excellent accuracy in the prediction of wave height the predicted wave direction, however, was not good. Although the last four models (i.e., elliptic or hyperbolic model) are capable of simulating strong wave diffraction, reflection, and resonance, those capabilities were not compared because RCPWAVE, Ref/Dif-1, and the physical model experiment are only capable of simulating water wave shoaling, refraction, and weak diffraction. Experimental data for waves traveling across an elliptic shoal were used as a standard for comparison.

Six numerical models: (l)RCPWAVE, (2)Ref/Dif-1, (3)RDE, (4)PBCG, (5)PMH, and (6)MIKE 21's EMS module, were examined for their performance on the simulation of water wave shoaling, refraction, and diffraction.


 0 kommentar(er)
0 kommentar(er)
